Answer:
13π square centimeters.
Step-by-step explanation:
• The radius of the circle centre O = 6cm
,
• The central angle, α = 130°
The shaded region is a sector.
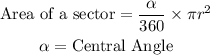
Therefore, the area of the shaded region is:
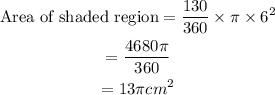
The area of the shaded region is 13π square centimeters.