Question

A food magazine published a listing of local restaurant ratings. Restaurant ratings 8 8 8 23 23 23 23 36 36 36 X is the rating that a randomly chosen restaurant was rated. What is the expected valu Write your answer as a decimal.

Answer 1

To solve this question, first we need to start building the probability distribution table, taking into account that the possible X are 8, 23 and 36. and that there were 10 ratings.

The probability distribution should look like this

Using the probability distribution apply the formula for the expected value

`E(x)=\sum ^n_(i\mathop=1)(x_i)(p(x_i))`

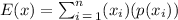
according to this the expected value is

`\begin{gathered} E(x)=8\cdot(3)/(10)+23\cdot(4)/(10)+36\cdot(3)/(10) \\ E(x)=(24)/(10)+(92)/(10)+(108)/(10) \\ E(x)=(224)/(10) \\ E(x)=22.4 \end{gathered}`

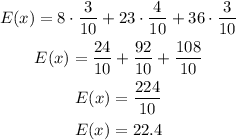
The expected value is 22.4