Given:
The equation of a first line is,
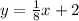
The objective is to find the equation of a second line perpendicular to the first line through the point (-8,0).
Step-by-step explanation:
The general equation of straight line is,

Here, m represents the slope of the line and b represents the y-intercept.
To find slope of first line:
By comparing the general equation with the first line,
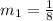
To find slope of second line:
For perpendicular lines, the product of their slope values will be -1.
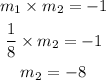
Since the second line passes throught the point(-8,0), the equation of the line can be calculated using slope point formula of straight line.
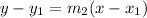
To find equation of the perpendicular line:
Consider the given points as,
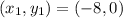
On plugging the values in the above equation,
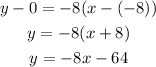
Hence, the equation of the perpendicular line is y = -8x - 64.