Solution:
The permutation formula is expressed as
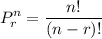
The combination formula is expressed as
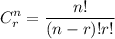
where
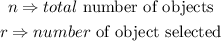
Given that 6 objects are taken at a time from 8, this implies that
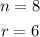
Thus,
Number of permuations:
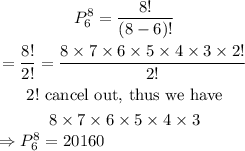
Number of combinations:
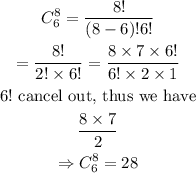
Hence, there are 28 combinations and 20160 permutations.