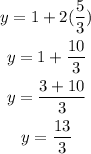Given the systems of equation
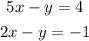
We want to solve it using the substitution method. The first step is to get one equation from the systems of equations and solve it in terms of x. I will choose the second equation in this case. We have
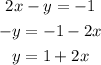
Put this equation on the first equation present in the system of equations and solve for x. We have
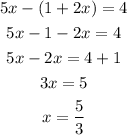
Use this value of x and substitute it on one of the equations on the system of equations to solve for the value of y. In this case, I will be using equation 2. We have