Question

Distributive Property 16. Which expression shows how to use the Distributive Property to solve 4 x 221? A. (4 x 200) x (4 x 20) x (4 x 1) B. (4 + 200) + (4 + 20) + (4 + 1) c. (4 x 200) + (4 x 20) + (4x1) D. (4x2) + (4 x 2) + (4 x 1)

Answer 1

According to Distributive property, you know that:

`\begin{gathered} a(b+c)=ab+ac \\ a(b-c)=ab-ac \end{gathered}`

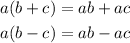
In this case, you need to solve the following multiplication:

`4\cdot221`

One of the ways to solve it is using the Distributive property. Notice that:

- The first digit 2 is located in the hundreds place.

- The second digit 2 is located in the tens place.

- The last digit 1 is located in the ones place.

Then, based on the above, you can set up the following expression:

`(4\cdot2\cdot100)+(4\cdot2\cdot10)+(4\cdot1)`

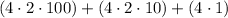
Multiplying the number inside the parentheses and adding the products, you get:

`(4\cdot200)+(4\cdot20)+(4\cdot1)=800+80+4=884`

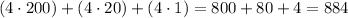
The answer is: Option C.