The SOLUTION
Recall the formula for compound interest formula

For the amount to double then A=2P
From the question it follows:
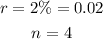
Substituting these values gives:
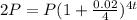
Solve for t
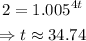
Therefore the number of years it will take for the amount to double is 34.74 years
Using the compounded continuously formula
Substituting values gives
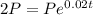
Solve for t
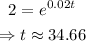
For compounded continuously, the investment will double in about 34.66 years