The given inequality is 2x-3y < 5.
To find the ordered pairs that satisfy the inequality, we have to evaluate it.
For (2,0).
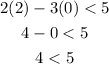
Given that 4<5 is true, we can conclude that (2,0) satisfies the inequality.
For (1,-1).
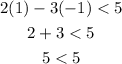
The statement 5<5 is false. So, (1,-1) does not satisfy the inequality.
For (0,0).

The statement 0<5 is true. So, (0,0) is a solution to the given inequality.
For (2,4).
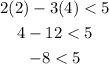
Given that -8<5 is correct. (2,4) satisfies the inequality.
Therefore, the solutions are (2,0), (0,0), and (2,4).