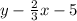Two lines are parallel if they have the same slope and the are perpendicular if m_2 = -1/m_1.
We first write the three lines in slope-intercept form:
Line 1:
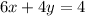
subtracting 6x from both sides gives
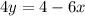
Dividing both sides by 4 gives
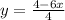
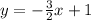
Line 2:
Dividing both sides by 3 gives
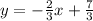
Line 3:
This equation is already in slope-intercept form: