Question

A committee of 4 is being formed randomly from the employees at a school: 6 administrators, 37 teachers and 4 staff. What is the probability that all 4 members are teachers? Express your answer as a fraction.

Answer 1

Given:

The number of administrators is a = 6.

The number of teachers is t = 37.

The number of staff is s = 4.

The number of employees required for the committee is r = 4.

The objective is to find the probability that all 4 members are teachers.

Step-by-step explanation:

The total number of members is,

`\begin{gathered} n=a+t+s \\ =6+37+4 \\ =47 \end{gathered}`

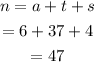
The number of ways in selecting 4 members out of 47 will be,

`\begin{gathered} ^(47)C_4=(47!)/((47-4)!4!) \\ =(47*46*45*44*43!)/(43!4*3*2*1) \\ =178365 \end{gathered}`

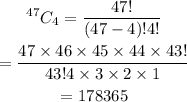
Now, the number of ways in selecting 4 members from 37 teachers will be,

`\begin{gathered} ^(37)_{}C_4=(37!)/((37-4)!4!) \\ =(37*36*35*34*33!)/(33!4*3*2*1) \\ =66045 \end{gathered}`

To find the probability:

Now, the probability of forming all 4 members as teachers will be,

`\begin{gathered} P=(66045)/(178365) \\ =(4403)/(11891) \end{gathered}`

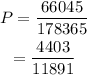
Hence, the probability that all 4 members are teachers is 4403/11891.