Question

A person standing on a moving walkway travels 80 feet in 40 seconds. What equation represents this relationship? How many feet will the person travel in 10 secondsA. y=4x ; 40 feetB. y = 2x ; 2 feetC. y = 1/2x ; 5 feetD. y = 2x ; 20 feet

Answer 1

`y=2x;y=20\text{ feet}`

Step-by-step explanation

We want to find the equation that represents the relationship between the distance moved and the time spent.

This relationship is a proportional relationship.

The general form of a proportional relationship is given as:

`\begin{gathered} y=kx \\ \text{where k = constant of proportionality} \end{gathered}`

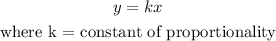
Since distance is proportional to time, we have that travelling 80 feet in 40 seconds will mean:

`\begin{gathered} 80=k\cdot40 \\ k=(80)/(40) \\ k=2\text{ ft/sec} \end{gathered}`

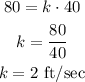
Therefore, the equation is:

`y=2x`

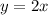
To find the distance travelled in 10 seconds, we have to find y when x is 10.

That is:

`\begin{gathered} y=2\cdot10 \\ y=20\text{ feet} \end{gathered}`