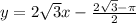Question

Find an equation of the tangent line to the graph of the function at the given point.y=3arcsinx, (1/2,pi/2)

Answer 1

Given:

`y=3arcsin(x)`

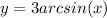
Let's find the equation of the tangent line over the interval:

`((1)/(2),(\pi)/(2))`

First find the derivative of the equation:

`y^(\prime)=(3)/(√(1-x^2))`

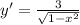
Now evaluate the derivative when x = 1/2:

`\begin{gathered} y^(\prime)=\frac{3}{\sqrt{1-((1)/(2))^2}} \\ \\ y^(\prime)=\frac{3}{\sqrt{1-(1)/(4)}} \\ \\ y^(\prime)=\frac{3}{\sqrt{(3)/(4)}} \\ \\ \end{gathered}`

Solving further:

`\begin{gathered} y^(\prime)=(3)/((√(3))/(√(4))) \\ \\ y^(\prime)=(3√(4))/(√(3)) \\ \\ y^(\prime)=(3*2)/(√(3)) \\ \\ y^(\prime)=(6)/(√(3)) \\ \\ y=(6)/(√(3))*(√(3))/(√(3)) \\ \\ y=(6√(3))/(3) \\ \\ y=2√(3) \end{gathered}`

Now, the slope of the tangent line is 2√3.

Input the value for the slope, then put (1/2, π/2) for x1 and y1 in point-slope form:

`\begin{gathered} y-y1=m(x-x1) \\ \\ y-(\pi)/(2)=2√(3)(x-(1)/(2)) \\ \\ y-(\pi)/(2)=2√(3)x-2√(3)*(1)/(2) \\ \\ y-(\pi)/(2)=2√(3)x-√(3) \end{gathered}`

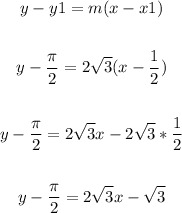
Now add π/2 to both sides:

`\begin{gathered} y-(\pi)/(2)+(\pi)/(2)=2√(3)x-√(3)+(\pi)/(2) \\ \\ y=2√(3)x-(2√(3)-\pi)/(2) \end{gathered}`

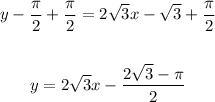
Therefore, the equation of the tangent line is:

`y=2√(3)x-(2√(3)-\pi)/(2)`

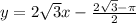
ANSWER:

`y=2√(3)x-(2√(3)-\pi)/(2)`