Answer:
D. 3.5
Step-by-step explanation:
A fair six-sided dice has faces labeled 1,2,3,4,5 and 6.
The probability of each of the faces occurring = 1/6
Thus, the expected value for a fair six-sided dice roll is:
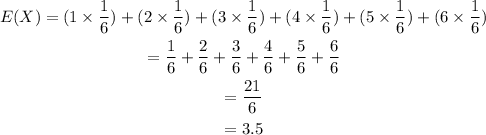
The expected value for a fair six-sided dice roll is 3.5.