Question

Help me solve this problem! Thank you! Only answer if you understand

Answer 1

Composite transformation:

`(D_2\circ T_(<-5,-3>))`

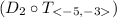
1. Translation 5 units to the left and 3 units down:

`(x,y)\rightarrow(x-5,y-3)`

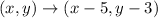
Apply the rule above to vertices of given triangle:

`\begin{gathered} M(3,5)\rightarrow M^(\prime)(3-5,5-3) \\ M^(\prime)(-2,2) \\ \\ \\ N(-1,4)\rightarrow N^(\prime)(-1-5,4-3) \\ N^(\prime)(-6,1) \\ \\ \\ O(1,8)\rightarrow O^(\prime)(1-5,8-3) \\ O^(\prime)(-4,5) \end{gathered}`

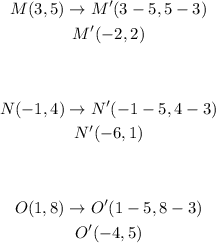
2. Dilation with factor 2:

`(x,y)\rightarrow(2x,2y)`

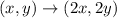
Apply the rule above to vertices M'N'O':

`\begin{gathered} M^(\prime)(-2,2)\rightarrow M^(\prime)^(\prime)(2*-2,2*2) \\ M^(\prime)^(\prime)(-4,4) \\ \\ N^(\prime)(-6,1)\rightarrow N^(\prime)^(\prime)(2*-6,2*1) \\ N^(\prime)^(\prime)(-12,2) \\ \\ O^(\prime)(-4,5)\rightarrow O^(\prime)^(\prime)(2*-4,2*5) \\ O^(\prime)^(\prime)(-8,10) \end{gathered}`

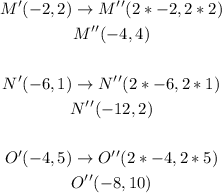
Then, the vertices of image after the composite transformation are:

M''(-4,4)

N''(-12,2)

O''(-8,10)

Graph: