Given the points : ( 3 , 8 ) and ( -2 , 5 )
We need to find the coordinates of the point equidistant from the given point and should be on the y - axis
as the point will be on y - axis : the x - coordinates of the point will be 0
So, let the coordinates of the point is ( 0 , a )
The distance between any two points is given by the formula :
![d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}](https://img.qammunity.org/2023/formulas/mathematics/college/be685jmxw05hm2tq94m5iuge2xjynn1hfn.png)
So, the distance between ( 3 , 8 ) and ( 0 , a ) will be :
![d_1=\sqrt[]{(0-3)^2+(a-8)^2}=\sqrt[]{9+(a-8)^2}](https://img.qammunity.org/2023/formulas/mathematics/college/g9gnrc74sdzkji5g2o7w5szp3jg8t1tirr.png)
And the distance between ( -2 , 5 ) and ( 0 , a ) will be :
![d_2=\sqrt[]{(0+2)^2+(a-5)^2}=\sqrt[]{4+(a-5)^2}](https://img.qammunity.org/2023/formulas/mathematics/college/70banl8cts2x99v9la25r8whxhllt18obx.png)
the two distances are equal
![\begin{gathered} d_1=d_2 \\ \sqrt[]{9+(a-8)^2}=\sqrt[]{4+(a-5)^2} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/9y6a4ykrfwrsio40n7vgjd92chyvzxj94y.png)
squaring both sides then solve for a:
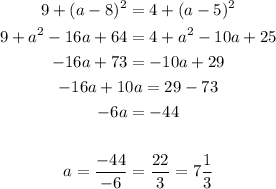
so, the answer is the coordinates of the point is ( 0 , 7 1/3 )