Question

Consider the following functions.f(x) = x2 - 6x + 9 and g(x) = x - 3Step 1 of 2: Find(9)«. .(x). Simplify your answer.Answer(96) =

Answer 1

Given the functions

`\begin{gathered} f(x)=x^2-6x+9 \\ g(x)=x-3 \end{gathered}`

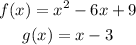
You have to find the quotient between both functions, that is (f/g)(x)

`((f)/(g))(x)=(x^2-6x+9)/(x-3)`

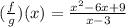
To solve this division, the first step is to factor the numerator.

To factor the quadratic function, you have to find a value or values whose sum is -6 and their product is 9.

The number that fulfills both characteristics is -3

The factor of f(x) is (x-3) and its factorized form is:

`\begin{gathered} f(x)=x^2-6x+9 \\ f(x)=(x-3)^2 \end{gathered}`

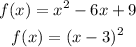
You can rewrite the quotient as follows:

`\begin{gathered} ((f)/(g))(x)=(x^2-6x+9)/(x-3) \\ ((f)/(g))(x)=((x-3)^2)/(x-3) \end{gathered}`

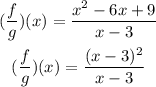
The next step is to simplify the expression:

`\begin{gathered} ((f)/(g))(x)=\frac{(x-3)^{\bcancel{2 }}}{\bcancel{x-3 }} \\ ((f)/(g))(x)=x-3 \end{gathered}`

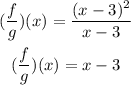
The result is (f/g)(x)=x-3