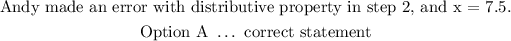We will investigate the laws associated with algebraic manipulations of numbers.
There are a total of three Laws asscoiated with the algebraic operators as follows:

Commutative Law:
This law pertains to the order in which a addition " + " and multiplication " x " operators are applied on two numbers.
Lets say we have two numbers named ( a ) and ( b ). The commutative law representing additon would be:
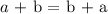
The multiplication operator would be:
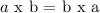
We see that exchange of numbers does not change the result of both addition and multiplication.
Associative Law:
This law pertains to the application of an addition " + " and multiplication " x " operators in groups. The grouping of numbers is represented by a parenthesis " ( ) ".
Lets say we have three numbers ( a , b and c ). We can have three possible combinations of grouping with each operator as follows:
Addition:
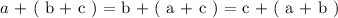
Multiplication:

We see that each group of combination above for both addition and multiplication leads to the same result disregarding which pair is operated first!
Distributive Law:
This law pertains to an application of addition operator on two element group which is multiplied by a third number.
Using the same three numbers ( a,b, and c ) we have:
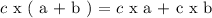
The above represents the solving of parenthesis by removing the round brackets " ( ) " and applying the multiplication operator with each element in the group.
Andy tries to solve an equation as follows:
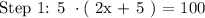
To remove the parenthesis on the left hand side of the " = " sign we need to apply the distributive law.
The next step involves the application of distributive law as follows:

Therefore, we see that Andy applied the distributive law incorrectly in step 2. Where he applied the multiplication operator with just the first element of the group i.e ( 2x ) and left out on the second one i.e ( 5 ).
We will continue forward with the next step after applying the distributive law correctly. We will try to isolate the term involving the variable ( x ) by subtracting 25 on both sides of the " = " sign as follows:

Then we will divide the entire equation by the multiple of the variable ( x ) as follows:
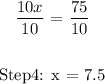
Therefore, the correction statement to Andy's solution would be: