A dilation with a scale factor of k=1/3 with the center of dilation at the origin will perform the following transformation in any point (x,y):
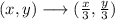
We have a triangle with vertices U, V and W.
We apply the transformation to these points and get:
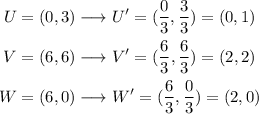
Answer: U'=(0,1), V'=(2,2), W'=(2,0)
The right option is Option D.