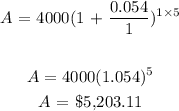Question

Find the amount in a continuously compounded account for the following condition.Principal, $4000; Annual interest rate, 5.4%; time, 5 yearswhat is the amount after 5 years? round to nearest cent as needed round all intermediate values to five decimal places as needed

Answer 1

`A\text{ = \$5,203.11}`

Step-by-step explanation:

Here, we want to get the amount in a continuous compounding fashion

We have the formula to use as:

`A\text{ = P(1 + }(r)/(n))^(nt)`

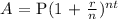
where:

A is the amount after 5 years

P is the principal which is $4,000

r is the interest rate which is 5.4% = 5.4/100 = 0.054

n is the number of times interest is compounded which is 1 (annual)

t is the time which is 5 years

Thus, we have the amount calculated as follows:

`\begin{gathered} A\text{ = 4000(1 + }(0.054)/(1))^(1*5) \\ \\ A=4000(1.054)^5 \\ A\text{ = \$5,203.11} \end{gathered}`