Question

Given two terms in a geometric sequence find the explicit formula

Answer 1

Option C)

Step-by-step explanation:

To know which is the explicit formula we will replace n = 2 and n = 5. If we get 6 and 162 respectively, we can say that it is the correct formula.

For option A)

`\begin{gathered} a_n=(2)/(15)\cdot5^(n-1) \\ \\ \text{ If n = 2} \\ a_2=(2)/(15)\cdot5^(2-1)=(2)/(15)\cdot5^1=(2)/(15)\cdot5=(2)/(3) \end{gathered}`

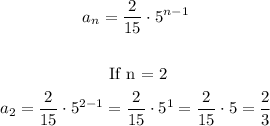
Since 2/3 is different from 6, we get that this is not the correct answer

For option B)

`\begin{gathered} a_n=(2)/(3)\cdot5^(n-1) \\ \\ a_2=(2)/(3)\cdot5^(2-1)=(2)/(3)\cdot5=(10)/(3) \end{gathered}`

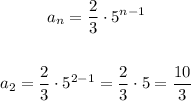
Since 10/3 is different from 6, we get that this is not the correct answer

For option C)

`\begin{gathered} a_n=2\cdot3^(n-1) \\ a_2=2\cdot3^(2-1)=2\cdot3^1=6 \\ a_5=2\cdot3^(5-1)=2\cdot3^4=2\cdot81=162 \end{gathered}`

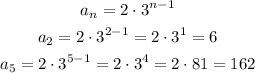
Therefore, the answer is option C)