The given polynomial is,
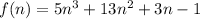
According to the factor theorem, x-a is factor of the polynomial f(x) only if f(a)=0.
Hence, n-2 is a factor of polyynomial f(n), then f(2) should be equal to zero.
So, first find f(2).
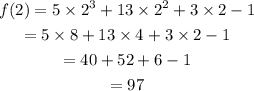
So,
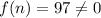
Therefore, x-2 is not a factor of the given polynomial