We have that the formula for the compound interest semi annually is:
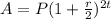
where A is the total amount, P is the principal amount, r is the interest rate and t is the time in years.
In this case, we have the following information:
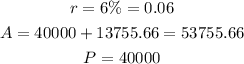
then, using the first equation, we get:

using logarithm on both sides of the equation, we get the following:
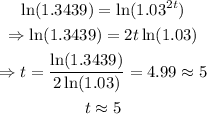
therefore, it wil take approximately 5 years to get the desired interest.