Question

Figure 1Figure 2Figure 3400-400-400-300-300-300-200+200-+2004100.100-100-.1011हैy=-0.2x²+21.2x-7.7y=0.4x² + 8.9x + 14.2y=0.7x² +0.5x +11(a) Which curve fits the data best?O Figure 1 Figure 2 Figure 3(b) Use the equation of the best fitting curve from part (a) to predict the speedof the object at 6 seconds. Give an exact answer, not a roundedapproximation.meters per second

Answer 1

a) Line or curve of best fit refers to a line or curve through a scatter plot of data points that best expresses the relationship between those points, in other words, it is the line or curve that is closest to the given set of data values.

So from our above definition of the line or curve of best fit, the figure that best represents the curve that is closest to the given set of data values scatter around the plot is figure 2.

For Figures 1 and 3, the curves drawn there are too far from the data set, hence they cannot be the curve that fits the data best.

b) From (a) , our best curve is figure 2 represented by the equation below

`y=0.7x^2+0.5x+11^{}^{}`

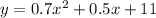
To predict the speed at 6 seconds means we will insert x=6 into the equation above, the answer we get is our speed.

`\begin{gathered} y=0.7x^2+0.5x+11 \\ substitute\text{ x=6 into the above equation} \\ y=0.7(6)^2+0.5(6)\text{ + 11} \\ y=\text{ (0.7 x 36) + 3 +11} \\ y\text{ = 25.2 + 3 + 11} \\ y=\text{ 39.2} \end{gathered}`

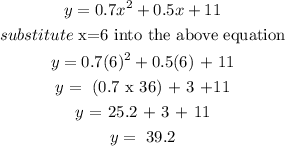
The speed is 39.2 meters per second