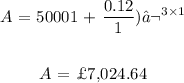Answer:
Step-by-step explanation:
Here, we want to get the value of the deposit after the stipulated time
Mathematically:
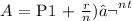
where:
A is the amount after the given time
P is the deposited amount which is 5,000 pounds
r is the interest rate which is 12% = 12/100 = 0.12
n is the number of times interest is compounded yearly which is 1
t is the number of years
i) One year
We have that as:
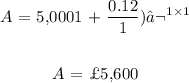
ii) Three years