ANSWER
• x = -2
,
• x = 10
Step-by-step explanation
Let 'x' be the number. The square of a number is x². Then that equals to 8 times the number (8x) plus 20. Therefore we have the equation
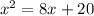
We can rewrite this equation as follows:

And use the quadratic formula to determine the number:
![x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}](https://img.qammunity.org/2023/formulas/mathematics/college/rxvf73usjbbwyik14knxdemoz21vfz2ufc.png)
In this case, a = 1, b = -8 and c = -20:
![x=\frac{8\pm\sqrt[]{8^2-4\cdot1\cdot(-20)}}{2\cdot1}](https://img.qammunity.org/2023/formulas/mathematics/college/q6k9j80v38vsu9l0llawio7s7f2dhboheq.png)
![x=\frac{8\pm\sqrt[]{64^{}+80}}{2}](https://img.qammunity.org/2023/formulas/mathematics/college/w4bfwos21js3pc1paoaked34hyd8rlsel2.png)
![x=\frac{8\pm\sqrt[]{144}}{2}](https://img.qammunity.org/2023/formulas/mathematics/college/ghos5m6bgf88lzpagf00zf8oq91poppgea.png)
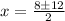
We have two posible results for this number. One when subtracting 12:
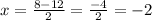
and the other when adding 12:

So the number is either -2 or 10.