Question

4.5 Quadratic Application Word Problemsa1. Jason jumped off of a cliff into the ocean in Acapulco while vacationing with some friends. Hisheight as a function of time could be modeled by the function h(t)=-16t? +16t+480, where t isthe time in seconds and h is the height in feet.a. How long did it take for Jason to reach his maximum height?5 secondsb. What was the highest point that Jason reached?I484 feetc. What is Jason’s initial height?

Answer 1

Case: Quadratic Application Word Problem

Given:

`h(t)=-16t^2+16t+480`

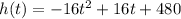
Required:

a. How long did it take for Jason to reach his maximum height?

b. What was the highest point that Jason reached?

c. What is Jason’s initial height?

Method:

Step 1: How long did it take for Jason to reach his maximum height?

To calculate this, we find the vertex

`\begin{gathered} h(t)=-16(t^2-t-30) \\ h(t)=-16[(t^2-t+(1)/(4)-(1)/(4)-30) \\ h(t)=-16[(t-(1)/(2))^2-(1)/(4)-30) \\ h(t)=-16(t-(1)/(2))^2+4+480 \\ h(t)=-16(t-(1)/(2))^2+484 \end{gathered}`

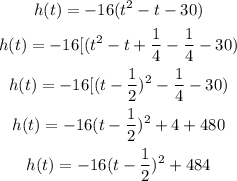
From here, the vertex is at (1/2, 484).

Hence it takes 1/2 a second to reach the maximum height

Step 2: What was the highest point that Jason reached?

Also, from the vertex, we get the highest height reached

The maximum height reached was 484 feet

Step 3: What is Jason’s initial height?

The initial height is gotten at the start of the motion, i.e. h(0) = ?

`\begin{gathered} h(t)=-16t^2+16t+480 \\ h(0)=480 \end{gathered}`

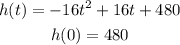
Hence the initial height was 480 feet

Final answer:

A) Time = 1/2 second

B) Maximum Height, H= 484 feet

C) Initial Height, H= 480 feet