Question

A 20.0 m long string of Christmas lightshas two wires twisted around each other0.00234 m apart. One carries 3.47 A ofcurrent toward the tree and the othercarries the same current away from thetree. What is the (+) magnitude of themagnetic force between the wires?[?] N

Answer 1

Given:

• Length of wire, L = 20.0 m

,

• Distance between wires, r = 0.00234 m

,

• Current carried by each wire I = 3.47 A

Let's find the magnitude of magnetic force between the wires.

To find the magnitude of magnetic force, apply the formula:

`F=(\mu_oI_1I_2L)/(2\pi r)`

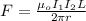
Where:

• μ₀ = 4π x 10⁻⁷ T m/A

,

• I1 = 3.47 A

,

• I2 = 3.47 A

,

• r = 0.00234 m

,

• L = 20.0 m

,

• F is the magnitude of magnetic force between the wires.

Plug in the values and solve for x:

`\begin{gathered} F=(4\pi*10^(-7)*3.47*3.47*20.0)/(2\pi *0.00234) \\ \\ F=(2*10^(-7)*240.818)/(0.00234) \\ \\ F=(4.81636*10^(-5))/(0.00234) \\ \\ F=0.02058\text{ N} \end{gathered}`

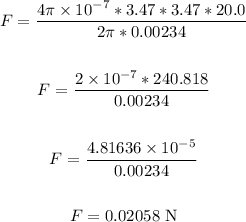
Therefore, the magnitude of magnetic force between the wires is 0.02058 N

• ANSWER:

0.02058 N