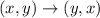Answer:
a)
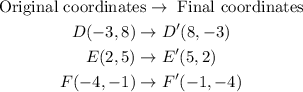
b)
Step-by-step explanation:
a)
The coordinates of the image on the graph are;
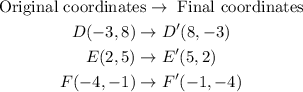
b)
From the solution in a above, we can derive the general rule of the reflection from triangle DEF to D'E'F';
From the solution in a above, the values of the coordinates of x and y were interchanged.
x to y and y to x to give the image.
So, we can write the general rule as;