Question

One of the walls of Georgia's room has a radiator spanning the entire lenght and she painted a mirror covering the portion of that wall above the radiator. Based on info below.- the volume of G room is 1296 cubic feet- the floor of G room is a square with 12 foot sides- radiator is 1/3 of the height of the roomwhat is the area in sq feet covered by G mirror?

Answer 1

Given:

Volume of room = 1296 cubic feet

One side of the square floor of the room = 12 ft

Radistor is ⅓ of the height of the room.

Let's find the area covered by the mirror.

Here, the room has the shape of a square prism.

To find the height of the room, apply the formula:

`V=a^2\ast h`

Where:

V = 1296 cubic feet

a = 12 ft

Let's solve for h:

`\begin{gathered} 1296=12^2\ast h \\ \\ 1296=144\ast h \\ \\ \text{Divide both sides by 144:} \\ (1296)/(144)=(144\ast h)/(144) \\ \\ 9=h \\ \\ h=9\text{ ft} \end{gathered}`

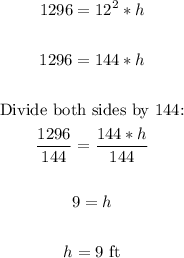
The height of the room is 9 feet.

Given that the radiator is ⅓ of the height of the room.

We have:

`\begin{gathered} (1)/(3)\ast h \\ \\ =(1)/(3)\ast9 \\ \\ =3\text{ ft} \end{gathered}`

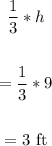
This means the height of the radiator is 3 ft

Let's find the area covered by the mirror.

Apply the area of a rectangle.

Since the radiator covers the entire length of the room, the length the radiator covers is 12 ft

The height of the radiator is 3ft

`\begin{gathered} A=3\ast12 \\ \\ A=36\text{ square fe}et \end{gathered}`

Therefore, the area covered by the G mirror is 36 square feet

ANSWER:

36 square feet