Question

hi I'm using goformative to answer my question, I will appreciate if I can get help with it, I will paste the image of my question

Answer 1

we have the point k(-2,4)

see the attached figure to better understand the problem

the point k lies in the II quadrant

`\cos (\theta)=-\cos (x)`

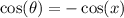
because angle theta and angle x are supplementary angles

cos(x)=x/c

where

c^2=2^2+4^2

c^2=20

c=2√5

so

cos(x)=2/2√5

cos(x)=1/√5

therefore

cos(theta)=-1/√5

simplify

cos(theta)=-√5/5