Equation: S (t)= -16t^2+960
A) The average rate of change of a function over an interval (a,b) is given by:
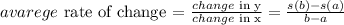
Then:

For s(4)
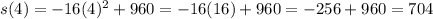
For s(1)
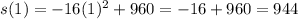
Therefore average rate of change is:
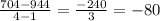
Answer: average rate of change = - 80 ft/s
B) This is just the value of the derivative when t = 3, so
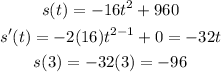
Answer: the instantaneous rate of change = - 96 ft/s
C) This is when s = 0, so:
![\begin{gathered} -16t^2+960=0 \\ -16t^2+960-960=0-960 \\ -16t^2=-960 \\ (-16t^2)/(-16)=(-960)/(-16) \\ t^2=60 \\ t=\sqrt[]{60} \\ t=7.75 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/a4ggq1jmhciown6cyr6n7m0lc1pcgvujrl.png)
Answer: time = 7.75 s
D) This happens when t = 7.75, so:

Answer: instantaneous rate of change of the dollar just before it hits the ground = - 248 ft/s