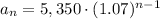The principal is $5,000.
The growth factor is 1.07.
To create the table, we have to multiply 1.07 by each value in $.
5,000 x 1.07 = 5,350 (1 year).
5,350 x 1.07 = 5,724.50 (2 years).
5,724.50 x 1.07 = 6,125.22 (3 years).
6,125.22 x 1.07 = 6,553.99 (4 years).
6,553.99 x 1.07 = 7,012.77 (5 years).
7,012.77 x 1.07 = 7,503.66 (6 years).
The table would be
Year Money
1 5,350
2 5,724.50
3 6,125.22
4 6,553.99
5 7,012.77
6 7,503.66
Given that this is a geometric sequence, we have
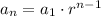
Where the first term is 5,350. Let's find the amount for n = 10.

After 10 years, we'll get $9,835.76.
Now, for n = 20.
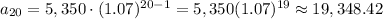
After 20 years, we'll get $19,348.42.
The exponential numbers for any given year would be