SOLUTION
To obtain the maximum value that she will jump, we will
1. Differentiate the given function
2. Equate it to 0, and obtain a value for x
3. Substitute the value of x back into the intial function and obtain what f(x) will be. The value that we obtain will be the maximum value.
Step 1:

Step 2:
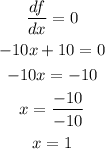
Step3:

Therefore the highest(maximum) point that Jane will reach in the air is 20feet.