Question

Oka-QQt40 ソニー万 10) -7x + 2y = 18 6x +6y=0 -x+2y=21 5*20 -7H2=18 - read 12) 3x – 5 y=17 -2 ya 3x+35e 3x+35=35-R-35 Tx+2y=-19 +84-5 SAS*=18 xes ADOR 13) -7x+4y=24REST 2zy 227 yeye 192y = -19*=-347 yup24 Solve each system by elimination. 15) 8x-6y=-20 -16x + y = 30 4x - 4y=0 3 x=-21+2.8 x=-5 ne- Y Y=24 Xene 8 y=-7 3-5-1732-18%.e- 14) 48-y=20 - 2x - 2y=10 j=13-20-12-26 EUX-20 4-214-2020 -25-EHO =10 2x-gxto 40 16) 6 - 12 = 24 y=43 -x-6y=4 Ex-by=4926 GX-129 =24 62-267 = 3+ 6x+-12y+-36)=-244247 Wizy-3y=48 * - 2 GX-12-24 16% -12y=0 -16x-12fe 30 Bye-16 2 %12.2016 -lax - 24 1 2

Answer 1

We have the following system of equations:

`\begin{gathered} -16+20x-8y=0 \\ 36=-18y-22x \end{gathered}`

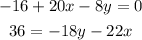
By multipliying the first equation by 22 and the second equation by 20, we get

`\begin{gathered} -352+440x-176y=0\ldots(A) \\ 720=-360y-440x\ldots(B) \end{gathered}`

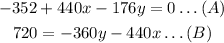
By substracting equation A and equation B, we get

`-352-720+440x-176y=360y+440x`

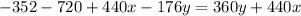
which gives

`-1072+440x-176y=360y+440x`

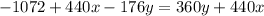
since we have 440x in both side, we can cancel out them. then we have

`-1072-176y=360y`

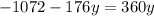
By moving -176y to the right hand side, we have

`-1072=360y+176y`

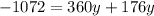
which gives

`-1072=536y`

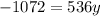
then, y is given by

`\begin{gathered} y=(-1072)/(536) \\ y=-2 \end{gathered}`

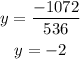
Now, in order to obtain x, we can substitute this result into our first equation. It yields,

`-16+20x-8(-2)=0`

which gives

`\begin{gathered} -16+20x+16=0 \\ 20x+0=0 \\ x=0 \end{gathered}`

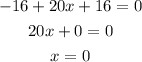
Therefore, the answer is x=0 and y= -2.