Question

20.Write and simplify the expression for the perimeter of the rectangle.D

Answer 1

`P=8b-2`

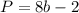
Step-by-step explanation

To find the perimeter of a rectangle, add the lengths of the rectangle's four sides.

so.

Step 1

Let

`\begin{gathered} Perimeter=lenght+length+width+widht \\ Perimeter=2\text{ lengths+2 widths} \\ Perimeter=2(\text{length}+\text{width)} \end{gathered}`

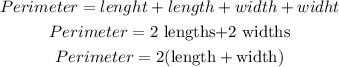
also, accordiing to the picture

`\begin{gathered} \text{length}=3b-1 \\ \text{width}=b \end{gathered}`

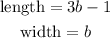
so, we need to replace to know the expression for the perimeter

Step 2

Replace,let permeter (P)

`\begin{gathered} Perimeter=2(\text{length}+\text{width)} \\ Perimeter=2(3b-1+b) \\ Perimeter=2(4b-1) \\ \text{apply distributive property to break the parenthesis} \\ Perimeter=2\cdot4b+2\cdot-1 \\ Perimeter=8b-2 \\ P=8b-2 \end{gathered}`

so, the answer is

P=8b-2

I hope this helps you