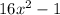Question

Find the product. (4x + 1)(4x - 1) =

Answer 1

To find the product of the following expression

`(4x+1)(4x-1)`

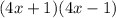
You have to distribute the multiplication between the parentheses terms, that is, multiply each term of the first parentheses by each term on the second parentheses.

You have to make a total of four multiplications:

`\begin{gathered} (4x\cdot4x)+(4x\cdot(-1))+(1\cdot4x)+(1\cdot(-1)) \\ 16x^2-4x+4x-1 \end{gathered}`

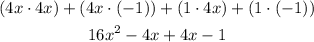
Once you had calculated all multiplications, order the like terms and simplify them, in this case, there are three types of terms, the x² term, the x term, and the constant.

The simplified result is:

`16x^2-1`