Question

What is the equation of this parabola in standard form?

Answer 1

The given information is:

- The vertex of the parabola is (6,2)

- The equation of its directrix is y=0

As the directrix is y=0, this means we have a vertical parabola.

The standard form of the equation of a vertical parabola is given by:

`(x-h)^2=4p(y-k)`

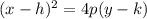
Where (h,k) is the vertex of the parabola, and p is given by the directrix equation:

`y=k-p`

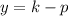
Let's start by finding p:

`\begin{gathered} (h,k)=(6,2) \\ h=6,k=2 \\ y=k-p \\ 0=2-p \\ \therefore p=2 \end{gathered}`

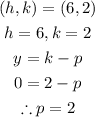
Now, replace the known values and find the equation:

`\begin{gathered} (x-6)^2=4*2*(y-2) \\ \therefore(x-6)^2=8(y-2) \end{gathered}`

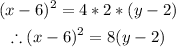
The answer is above.