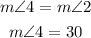So we know the value of:
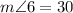
Let's start by calculating m2. For this, we have to assume that Broad Street and Monument Avenue are parallel.
Thus, m6 and m2 are Corresponding Angles, meaning they have the same measure. So:
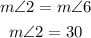
Now, since m1 and m2 complete each other to make a streight line, they are supplementary angles, so the add up to make 180°, thus:
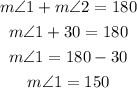
Similarly, we can see that m2 and m3 are also supplementary, thus:

Finally, m2 and m4 are Vertical Angles, meaning they have the same measure, so: