Question

supposed you wk 18.0 m straight west and then 25.0 m straight north. How far are you from your starting point, and what us the compass direction of a line connecting your starting point to your final position? (If you represent the two legs of the walk as vector displacements A and B, as in figure 3.53, then tbus problem asks you to find their sum R =A+B.)

Answer 1

To determine vector R we need to construct vectors A and B.

From the diagram we know that vectors A and B are:

`\begin{gathered} \vec{A}=-18\hat{i} \\ \vec{B}=25\hat{j} \end{gathered}`

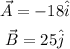
Then vector R is:

`\vec{R}=-18\hat{i}+25\hat{j}`

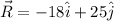
Now, that we have that we know that the magnitude of a vector is given as:

`R=\sqrt[]{R^2_x+R^2_y}^{}_{}`

where Rx and Ry are the components in each direction. With this in mind we have that the magnitude is:

`\begin{gathered} R=\sqrt[]{(-18)^2+(25)^2} \\ R=30.81 \end{gathered}`

Therefore, you are 30.81 meters from the starting point.

Now, to find the the direction (that is angle theta) we can use the following:

`\theta=\tan ^(-1)((R_y)/(R_x))`

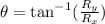
Now, from the figure we notice that we need to use the absolute value of each component (this means they both have to be positive) then we have:

`\theta=\tan ^(-1)((25)/(18))=54.25`

Therefore the direction is W52.25°N (this means that we measure the angle from west to north, as in the figure)