Question

A body starts with an initial velocity of 10m/s and moves along a straight line with a constant acceletion. When the velocity of the particle is 50m/s, the acceleration is reversed in direction. find the velocity of the particle when it reaches it's starting point.

Answer 1

Answer:

The velocity of the particle when it reaches the starting point = 70 m/s

Step-by-step explanation:

For the first part of the motion

The initial velocity, u = 10 m/s

The final velocity v = 50 m/s

`\begin{gathered} v^2=u^2+2as_1 \\ 50^2=10^2+2as_1 \\ 2500-100=2as_1 \\ 2as_1=2400..........(1) \end{gathered}`

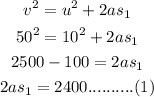
For the second part of the motion

The initial velocity, u = 50 m/s

Final velocity, v = 0 m/s

`\begin{gathered} v^2=u^2-2as_2 \\ 0=50^2-2as_2 \\ 2as_2=2500........(2) \\ where\text{ s}_1\text{ is the distance covered during the first part of the motion} \\ s_2\text{ is the distance covered during the second part of the motion} \\ \end{gathered}`

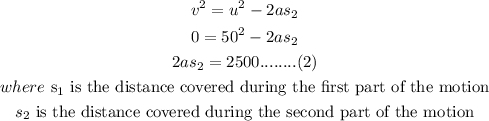
Add equations (1) and (2)

`\begin{gathered} 2as_1+2as_2=2400+2500 \\ 2a(s_1+s_2)=4900........(3) \end{gathered}`

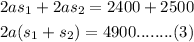
Taking the whole motion as a whole

Let the velocity of the particle when it reaches the starting point be vn

`\begin{gathered} v_n^2=u^2+2a(s_1+s_2) \\ \\ v_n^2=0^2+4900 \\ \\ v_n^2=4900 \\ \\ v_n=√(4900) \\ \\ v_n=70\text{ m/s} \end{gathered}`

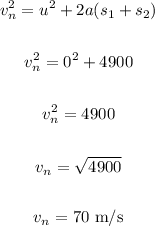
The velocity of the particle when it reaches the starting point = 70 m/s