Question

Cassy shoots a large marble (Marble A, mass: 0.06 kg) at a smaller marble (Marble B, mass: 0.03 kg) that is sitting still. Marble A was initially moving at a velocity of 0.7 m/s, but after the collision it has a velocity of −0.2 m/s. What is the resulting velocity of marble B after the collision? Be sure to show your work for solving this problem along with the final answer.I need to use this formaulam1u1 + m2u2 = m1v1 + m2v2and replace with this letterI had a tutor that explained to me very good , but me doing it byself I got lost

Answer 1

1.8 m/s

An

Step-by-step explanation:

We will use the given equation:

m1u1 + m2u2 = m1v1 + m2v2

Where m1 is the mass of the large marble, m2 is the mass of the smaller marble, u1 is the velocity of the large marble before the collision, u2 is the velocity of the smaller marble before the collision, v1 is the velocity of the large marble after the collision and v2 is the velocity of the large marble after the collision.

So, replacing the values, we get:

m1 = 0.06 kg

m2 = 0.03 kg

u1 = 0.7 m/s

u2 = 0 m/s

v1 = -0.2 m/s

Therefore:

`\begin{gathered} 0.06(0.7)+0.03(0)=0.06(-0.2)+0.03v_2 \\ 0.042=-0.012+0.03v_2 \end{gathered}`

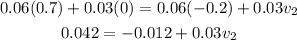
So, solving for v2, we get:

`\begin{gathered} 0.042+0.012=-0.012+0.03v_2+0.012 \\ 0.054=0.03v_2 \\ (0.054)/(0.03)=(0.03v_2)/(0.03) \\ 1.8m/s=v_2 \end{gathered}`

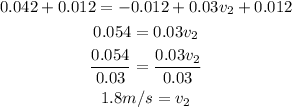
Then, the velocity of marble B is 1.8 m/s