Question

Which of the following is correct about the following functions 5x2+23x+12

Answer 1

We are given a quadratic equation to solve.

This requires our knowledge of factorization.

The factorization of the quadratic equation is given below:

`\begin{gathered} 5x^2+23x+12 \\ \text{This can also be written as} \\ \\ 5x^2+20x+3x+12 \\ \text{factorizing this} \\ 5x(x+4)+3(x+4) \\ \\ \text{factorizing even further} \\ (5x+3)(x+4) \end{gathered}`

To find the root of the quadratic equation, we equate the factorized form to zero

`\begin{gathered} (5x+3)(x+4)=0 \\ \text{If two numbers multiply to give zero, it means:} \\ \text{either one must be zero or both are zero} \\ \\ \therefore5x+3=0\text{ or} \\ x+4=0 \\ \\ \therefore x=-(3)/(5)\text{ or -4} \end{gathered}`

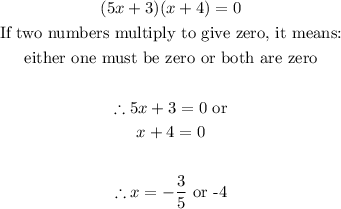
The factored form is:

(5x + 3)(x + 4)

The roots are:

x = -3/5 or x = -4