Question

The radius of a circle is 9 inches. What is the length of a 45° arc?

Answer 1

Step 1:

In this question, we are given the following:

The radius of a circle is 9 inches.

What is the length of a 45° arc?

Step 2:

The details of the solution are as follows:

`\begin{gathered} Arc\text{ length = }(\theta)/(360^0)\text{ x 2}\pi r \\ where\text{ }\theta\text{ = 45}^0 \\ Radius\text{ = 9 inches} \\ \end{gathered}`
`\begin{gathered} Arc\text{ length =}(45^0)/(360^0)\text{ x 2 x }\pi\text{ x 9} \\ =(1)/(8)\text{ x 2 x }\pi\text{ x 9} \\ =\text{ }(18\pi)/(8) \\ =\text{ }(9\pi)/(4) \\ =\text{ 7.068583471} \\ \approx\text{ 7.07 inches \lparen correct to 2 decimal places\rparen} \end{gathered}`

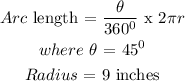
CONCLUSION:

The length of the Arc = 7.07 inches ( correct to 2 decimal places )