Answer:
Step-by-step explanation:
Given:
The equation describing the forest wood biomass per hectare as a function of plantation age t is:
y(t) = 5 + 0.005t^2 + 0.024t^3 − 0.0045t^4
The equation that describes the annual growth in wood biomass is:
y ′ (t) = 0.01t + 0.072t^2 - 0.018t^3
To find:
a) The year the annual growth achieved its highest possible value
b) when does y ′ (t) achieve its highest value?
a)
To determine the year the highest possible value was achieved, we will set the derivative y'(t) to zero. The values of t will be substituted into the second derivative to get the highest value
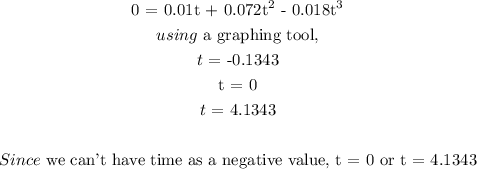

SInce t = 4.13, gives y ′' (t) = -0.316 (< 0). This makes it the maximum value of t
The year the annual growth achieved its highest possible value to the nearest whole number will be
year 4
b) y ′ (t) will achieve its highest value, when we substitute the value of t that gives into the initial function.
Initial function: y(t) = 5 + 0.005t^2 + 0.024t^3 − 0.0045t^4
![undefined]()