Given the points,
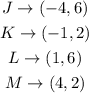
We need to solve for the distances JK,JL,KM,LM using the formula below,
![d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}](https://img.qammunity.org/2023/formulas/mathematics/college/be685jmxw05hm2tq94m5iuge2xjynn1hfn.png)
Firstly,
![\begin{gathered} JK=\sqrt[]{(-1-(-4))^2+(2-6)^2} \\ JK=\sqrt[]{(-1+4)^2+(-4)^2} \\ JK=\sqrt[]{3^2+16}=\sqrt[]{9+16} \\ JK=\sqrt[]{25}=5 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/kdj2o4ojabtnh8aub50ehs3ccx53x3lo06.png)
Secondly,
![\begin{gathered} JL=\sqrt[]{(1-(-4)^2+(6-6)^2} \\ JL=\sqrt[]{(1+4)^2+0^2}=\sqrt[]{5^2+0} \\ JL=\sqrt[]{25+0}=\sqrt[]{25}=5 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/u34sojk27a7dveicai6n522zj88vqbhx84.png)
Thirdly,
![\begin{gathered} KM=\sqrt[]{(4-(-1))^2+(2-2)^2} \\ KM=\sqrt[]{(4+1)^2+0}=\sqrt[]{5^2+0} \\ KM=\sqrt[]{25}=5 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/fuov8vq8q392zcef4sxk4ivpdenuianswn.png)
Lastly,
![\begin{gathered} LM=\sqrt[]{(4-1)^2+(2-6)^2} \\ LM=\sqrt[]{3^2+(-4)^2}=\sqrt[]{9+16} \\ LM=\sqrt[]{25}=5 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/emgebjz120bjepa0euxmfgqsyeryc8nagz.png)
Now, let us now calculate the slopes of line JK and LM,
The formula for the slope is given as,

Let us now solve for the slope of JK,
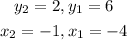
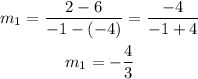
Let us now solve for the slope of LM,

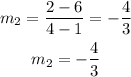
Properties of the shape
1) The two pairs of the opposite sides are parallel.
2) All the sides are equal.
Conclusively, the above properties of the shape tally with the properties of a rhombus.
Therefore, we can conclude that the name of the shape is a Rhombus.