Question

Use the graph f(x) = ex and the techniques of graphing to sketch h graph of each function. Determine the domain and the range of each function and indicate whether the function is increasing or decreasing. Also identify any horizontal asymptote. Explain in your own words the transformation of f (x). h(x) = 2 + 3e^−x

Answer 1

Horizontal asymptote: y=2

Reflect over the y-axis because of the negative raise power of x.

Domain: (-∞,∞)

Range: (2, ∞)

Decreasing all through the function.

Step-by-step explanation:

To approach this problem, we need to remember some of the transformation rules for functions:

*f(x)+d vertical translation up d units

*af(x) vertical stretch when a>1

Therefore, for the following function:

`h(x)=2+3e^(-x)_{}`

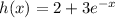
Since the function h(x) is shifted 2 units up, then the asymptote of the original Euler function would translate 2 units up.

Horizontal asymptote: y=2

Reflect over the y-axis because of the negative raise power of x.

Domain: (-∞,∞)

Range: (2, ∞)

Decreasing all through the function.