Question

WE’LL MATCH YOU WITH A TUTOR IN JUST A FEW SECONDS! thank you hle

Answer 1

Step-by-step explanation

If we solve the given equation for y, we have:

`\begin{gathered} y+2=0 \\ \text{ Subtract 2 from both sides} \\ y+2-2=0-2 \\ y=-2 \end{gathered}`

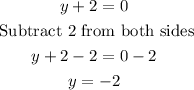
The above equation corresponds to the equation of a horizontal line whose y-coordinate is always -2.

The slope of a horizontal line is 0.

On the other hand, the y-intercept of a graph is the point where the graph crosses the y-axis. It is often referred to with just the y-value. In this case, we can see that the y-intercept of the line is -2.

Finally, we can see in the graph that the value of x is not always equal to the value of y.

Answer

The statement that is true about the line whose equation is y + 2 = 0 is:

The y-intercept is -2.