Recall the change of base formula of logarithms:
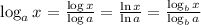
Note that here, b can be any base.
Using this property, it follows that the given expression can be written as:
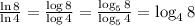
Hence, the first, second, and fourth choices are correct.
Other expressions are not equivalent expressions.
Choose the first, second, and fourth choices.