Given:
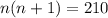
Sol:.
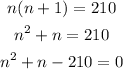
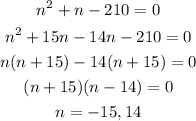
The product of two negative number that mean number is negative.
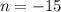
Then another number is:
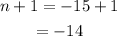
Number is -15,-14
Product of two negative consecutive number that mean if one number is "n" then another number is "n+1" and where n is a neagtive number so the value of "n" accept only negative value.
Consecutive number is define as a alternet number if the one number is "n" then another is may be "n+1" or "n-1".