
Given :-
A harmonic wave on a string is described by
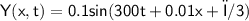
- x is in cm and t is in seconds
Answer 1 :-
Equation for travelling wave :-
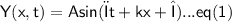
Equation for stationary wave :-
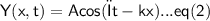
Given equation for wave :-
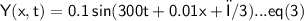
On comparing eq(1) , (2) and (3)
We can conclude that, Given wave represent travelling wave.
Answer 2 :-
From solution 1 , We can say that,
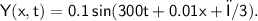
It is travelling from right to left direction
Hence, The direction of its propagation is right to left that is towards +x direction.
Answer 3 :-
Here, We have to find the wave period
We know that,
Wave period = wavelength / velocity
Wave equation :-
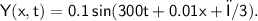
We know that,
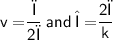
Subsitute the required values,



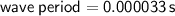
Answer 4 :-
The wavelength of given wave

Subsitute the required values,
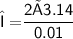
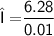
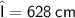
Answer 5 :-
We have wave equation

Travelling wave equation :-

Therefore,
Amplitude of the wave particle
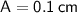
Hence, The amplitude of the particle is 0.1 cm